Bob Brown Cycles: analysis

Engineering:

Table of Contents:

Section II: Fillet Radius vs. Joint Strength

Material Myths

(Note: this page is meant to be a primer on tube deflection, not a thesis, for the complete story on all this consult an engineering text)

Chapter 1: Background

One all too common notion in the bicycling industry is that aluminum frames are inherently stiffer than steel frames. This page is here to show you some information on tubing stiffness, and what role geometric properties play in frame stiffness.

First off let's start with some definitions. Material strength is usually described as its Ultimate Tensile Strength or UTS. This is the maximum stress a material can withstand before failure. Sometimes Yield Strength is given for a material. This is the stress at which a material deviates from the proportional zone of the stress-strain curve(see fig.1). In other words, this is where plastic deformation begins. The Modulus of Elasticity (also referred to as Young's Modulus when in tension or compression) is a measure of the rigidity of the material. It is the ratio of stress to strain within the proportional area of the stress stain curve (see fig 1). For a complete explanation of all these terms refer to a reference text such as the ASM handbook or a Mechanics of Materials text.

Fig. 1

Chapter 2: FEA

Here are 4 FEA (Finite Element Analysis) models I created to show deflection for each tube. The deflections portrayed in each image are not to scale to each other, but the values listed in each table are absolute. Click on each image to view the full size image.

All models are of actual tube dimensions as given by the manufacturer. All tube models are fixed at one end, and have a 100 Lbf load applied downward to the other end.

	This is a model of a Reynolds 6061 Aluminum downtube. The diameter is 1.375" and the butt thicknesses are: 1.9/1.0/1.3mm This is a typical oversized Aluminum downtube. Maximum deflection is 1.6000" Mass is 240g
	This is a model of an oversized 6061 Aluminum downtube. The diameter is 1.5" and the butt profile is still 1.9/1/1.3mm. This represents an oversized and thick walled aluminum downtube. Maximum deflections is 0.8395" Mass is 309g.
	This is a model of a Reynolds 631 steel downtube. It's 1.25" in diameter, the butting is .9/.6/.9. This is a very common butt thickness for a non heat-treated tube. It is a very typical mid level steel downtube. Maximum deflection on this tube is 0.6718" Mass is 397g.
	This is model of a True Temper OX-Platinum downtube, 1.375" diameter. The butt thickness on this tube is .7/.4/.7 This is a fairly typical butt profile for top of the line steel tubing with a UTS around 180,000psi (OX-Gold, OX-Platinum, Reynolds 853, Columbus Nivacrom) Maximum deflection on this tube is 0.5339" Mass is 300g.

From these results it should be obvious that both material properties and geometric properties determine how stiff a frame is. Note that while the first aluminum tube is the lightest of the three, it's deflection is roughly three times that of the comparable steel tube. To prevent this, the aluminum tube would need to be larger diameter, or thicker wall, both of which increase mass. Even the 1.5" diameter aluminum tube has a deflection 1.5 times that of the True Temper tube. The overall result show the deflection to mass ratio to be better for both steel tubes than either aluminum tube. In fact, the model of the True Temper tube shown above shows less deflection and less mass than the 1.5" diameter aluminum tube.

A few other notes: The material's modulus of elasticity does not change significantly with alloying. Also it should be noted that neither heat treatment nor cold working alters the material's modulus of elasticity. The modulus is an inherent material property!

These results show that using round tubes, a steel frame can easily be made to be both stiffer and lighter than some aluminum frames. The drawback though is that even though the UTS of the new top-end steels is enough to support the bending loads, it's approaching the limit for buckling loads. In other words, you're more likely to have the tube buckle or dent than crack. This is a good reason why steel this thin should not be used on a frame that will see abuse such as a mountain or cyclocross frame, or even a larger sized road frame. They will dent easily, and are built with small safety factors. This is one reason why I mix and match tubing within or between sets. Different size and style frames need different types of tubes.

So draw what conclusions you want from this, the only real conclusion the data gives is that both materials have their good and bad points. Remember, these are only computer models of tubing under one form of stress. There are lots of other forces at work in a full bicycle frame in addition to bending, I used this as one example of one force.

Chapter 3: Deflection Tests

For this section, I performed a series of static deflection tests on similar frames constructed from different materials. The initial goal of this test was to determine some information about the stiffness to weight ratio of different rear triangle materials. I hear people commenting on how stiff, or how flexible their carbon fiber chainstays or seatstays make the bike feel, or how stiff their aluminum frame feels, this test was meant to test that notion. I hope to expand the test to front triangle stiffness soon as well.

The test consisted of mounting each frame in my alignment table, clamped by the BB shells. The alignment table is a 1" thick ground steel plate with a 2.5" diameter steel post mounted to it for the BB clamp. Any deflection in the table of fixture is negligible when considered next to the frame. All frames were clamped in the identical position with identical clamping force (applied via a torque wrench). A standard mass was placed on the drive side dropout and the chainstay deflection was measured using a dial indicator mounted to the alignment table. Each test was run 5 times with the values averaged afterwards. I was fortunate enough to have a number of similar sized high end road racing frames in my shop simultaneously for this test, including 3 top-of-their-line Bianchi road frames, one steel, one aluminum, and one full carbon fiber.

Results:

Bike	Material of Construction	Frame Weight	Measured Deflection
Bianchi XLEV2	7005 T-6 Aluminum	1127 g	0.030"
Bianch 928 (Luna)	Full Carbon Fiber	1270g	0.018"
Bianchi Martini Racing	Dedacchiai Steel Mix	1714g	0.023"
Bob Brown Cycles	True Temper OX-Platinum	1750g	0.024"
Klein Q-Pro	7005 main, Carbon seatstays	1235g	0.027"
Bob Brown Cycles	Columbus/Deda zero uno mix	1860g	0.019"
Salsa	Columbus Nivracrom .9/.6	1995g	0.017"

Obviously it would be helpful if one could know the exact wall thicknesses and butting on each of the tubes in these frames, but that isn't easily available. So the best we can do is interpret the data we have. That data falls in line with the FEA results from above, the aluminum frame has by far the greatest deflection. The heavier steel frames are stiffer. The full carbon Bianchi is quite stiff for it's weight, it's also on the heavier end when compared to other full carbon frames, but it still lighter than the other frames with similar stiffness. Hopefully I will be able to add more data to this table soon, as it will certainly help quantify the differences in materials.

Section II:

Fillet radius vs. joint strength

The following data was collected originally by Keith Bontrager. Please credit him if you cite any of this. What is here is a comparison of fillet radius vs. strength measured at several distances from the joint. It also shows a comparison of a tig welded joint to the fillet brazed joints. The data was provided by Keith in table form, I just arranged it to a plot-able format. Here is the plot showing his results.

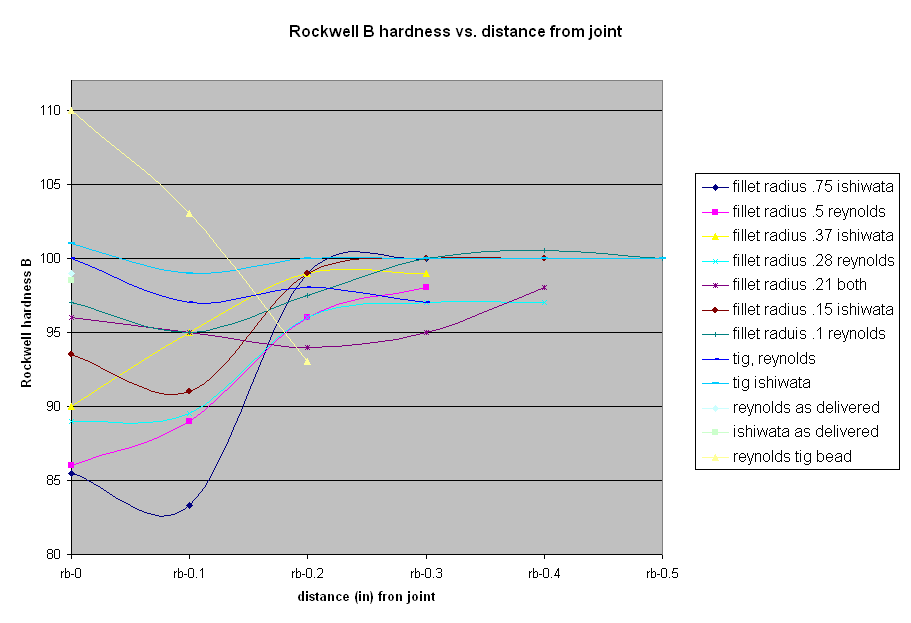
Chart Rockwell B
hardness vs. distance from joint

Rockwell hardness is nearly directly proportional to the tensile strength in these steels. It is a means to look at relative strength in the tubing at points very close to each other. The Ishiwata tubing was their Plain "CrMo" tubing, and the Reynolds was 531. All the dimensions are in inches, hardnesses are on the Rockwell B scale.

This data shows that as the fillet radius gets smaller, the initial strength of the material is higher, and the drop in the HAZ is less. It also shows the tig joints on both tubing had a higher initial strength and the least drop in the HAZ.

What this really shows is that the HAZ strength drop seems to be directly related to heat cycle time. The larger fillets require a longer heat cycle to form, smaller fillets have a shorter heat cycle, and the tig weld has the shortest. This does not mean that Fillet brazing is bad! In fact, the small fillets are not far off of the tig welded joint in strength drop. What is shows is that the builder should form the joint as quickly as possible yet still have full penetration. A less skilled tig weldor may take more time to complete the weld than Keith Bontrager did here, that would make the joint weaker than than one a skilled fillet brazed made. Also, running a second pass over a tig welded joint would greatly increase the heat cycle time, further weakening the tube. Many manufactures do this for cosmetic reasons, the result is a weaker joint. Again, the only result you can pull from this data is the skill of the builder has the most effect. If he/she can form the joint well in one quick pass, it will be a good joint regardless of method.

Section III:

Fatigue: how it works

Fatigue is a concept that is hard for many folks to grasp, but it's a very common failure mode in bicycles. Fatigue occurs when a material fails under a cyclical stress which is below the yield stress of the material. What is happening here is the material is repeatedly subject to an average stress which is lower than the materials yield stress. However there are localized regions of the body which are seeing localized stresses which exceed the yield stress. This can be due to surface irregularities, part geometry, or microscopic material defects, or even residual stress in the part left from manufacturing. At these regions of localized high stress, a micro crack develops because the material has exceeded it's yield strength locally. This micro-crack now forms a stress riser in the material, and on each repeated stress cycle, the crack grows. This will continue until the material fails and a viable crack forms.

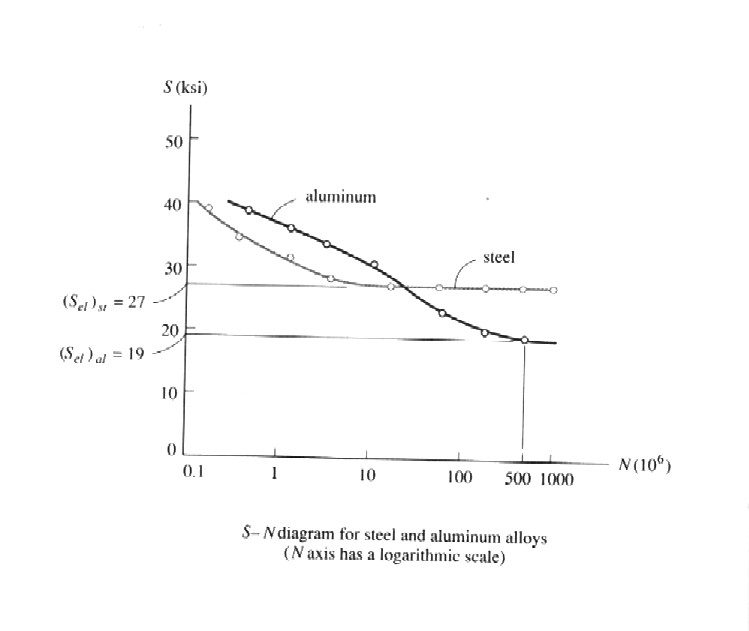
To avoid fatigue failures, the part should be designed with the endurance limit at the maximum stress level, not the yield stress. The endurance limit is the maximum stress at which a material will never exhibit fatigue, and it can be found from a materials stress-strain diagram (s-n diagram). Not all materials have an endurance limit, in this case, it is general practice to take the stress limit at 500 million cycles as the endurance limit. Below is a typical s-n diagram for steel and aluminum. You can see the steel curve bottoms out at about 27 ksi, this is the endurance limit for steel. The aluminum curve however does not bottom out, so for this material we take the endurance limit to be the stress at 500 million cycles, which is about 19 ksi. What this means is the designer should be sure the stress in a part is less than this endurance limit, and the part will not fatigue. For materials without a true endurance limit, the part will always eventually fatigue, however if properly designed, fatigue will not occur within the reasonable lifetime of the part.

(please ask permission if you wish to use any of this)